What is MOA? Understanding and using Minute of Angle
If you’re looking to understand Minutes Of Angle (MOA), you’re in luck! In this guide, we’re going to explore everything you need to know about Minute of Angle and how it relates to shooting a scoped rifle.
Understanding MOA will be key in learning how to zero a scope, make the right adjustments, and thus shoot more accurately. So, to help improve your understanding, we’ll start by looking at what exactly is MOA, how to use it and make the right adjustments, and give you important calculations and tables to use as well.
Table of contents
What is MOA?
MOA Definition: MOA stands for Minute of Angle and is commonly used with rifle scopes to help the shooter make adjustments to their scope so as to hit the target more accurately.
Now, there are a lot of parts to that, so let’s break this down starting with angles.
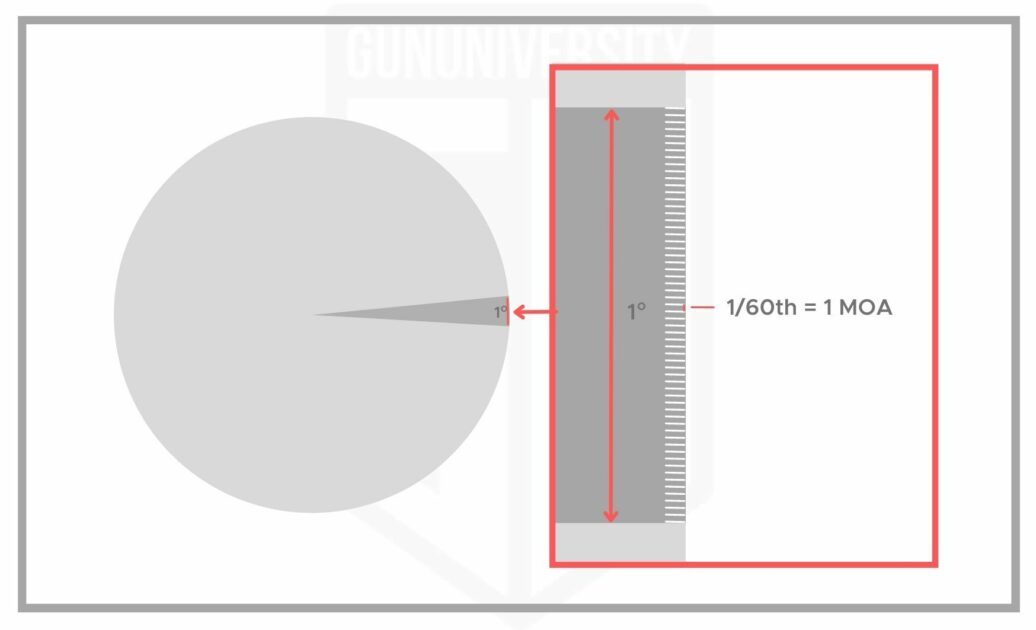
“Minute” is a way to refer to 1/60th of something. For example, 1 minute of an hour is 1/60th of that hour. Therefore, we now know that 1 MOA is one-sixtieth of an angle…but which angle is MOA referring to?
The “Angle” in MOA (Minute of Angle) is one of the 360 degrees in a circle. Therefore, MOA is 1/60th of one degree in a circle.
You may now be asking: “Why is MOA used with rifle scopes and long-range shooting?”
Great question! However, before we get into why (and how) MOA is used for shooting rifles, we need to first improve our understanding a bit more about what Minute of Angle is.
The first thing to understand about Minute of Angle (MOA) is that it is an angular and not a linear measurement.
That means a Minute of Angle is just like any other angular measurement… it can only refer to the angle between two things and NOT a length.
Imagine holding two laser pointers in your hand and pointing them both at the same spot on a target 100 yards away. Now, if you spread those two laser pointers apart at a certain angle, the lasers’ dots on the target will spread further apart.
If the laser pointers were spread apart at a 45-degree angle, for example, the dots would be very far apart at 100 yards. However, if the laser pointers were spread apart at a small angle, let’s say 1/60th of one degree (one MOA), then the laser pointers’ dots would be about 1 inch apart.
Pretty cool huh?
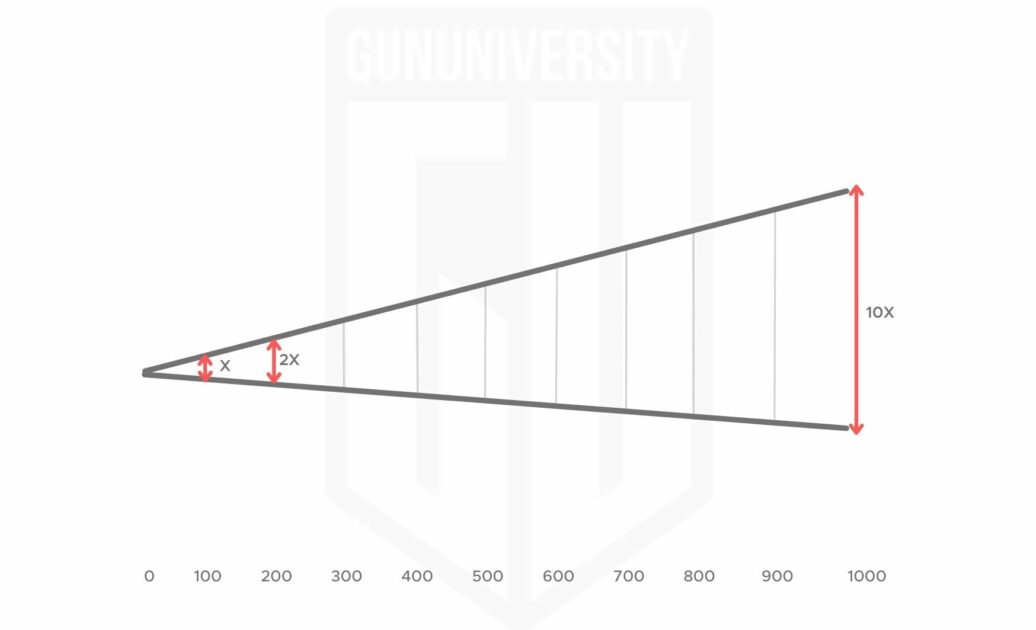
Now, even though we just learned that 1 MOA is about 1 inch at 100 yards, it is NOT accurate to say “1 MOA is an inch.” Technically, 1 MOA is precisely 1.047 inches per 100 yards. However, because Minute of Angle is an angular measurement, the linear distance (what we’re measuring in inches in this example with a ruler on the target) changes depending on how far away from the laser pointers we are. Therefore, it is more accurate to say that 1 MOA is about 1 inch at 100 yards.
Wait… did I just tell you to not worry about being precise when you’re trying to learn about precision shooting? Yes! Let me explain: At 1000 yards, a true Minute of Angle is 10.47 inches whereas my rounded MOA is 10 inches at 1000 yards.
I argue that the 0.47-inch difference in Minute of Angle calculations at 1000 yards is not enough to worry about. For one, the 0.47 inches is slightly wider than the width of the bullet you’re likely to be shooting at 1000 yards. Second, most shooters (me included) can not appreciate the benefit of a 0.47-inch bullet impact adjustment at 1000 yards because we can’t shoot groups that small.
Now, if you’re making multiple adjustments then that can start to add up. But in the grand scheme of things (or if you aren’t doing any precise pre-calculated shots), it doesn’t really matter.
Okay, so now that we know the nuance between MOA distances, here is a chart that shows the Minute of Angle for each of the distances. As you can see, its a linear growth as you move from 100 to 200, and so on.
| Distance | Inches in True MOA | Inches in Appx MOA |
|---|---|---|
| 100 | 1.047 | 1 |
| 200 | 2.094 | 2 |
| 300 | 3.141 | 3 |
| 400 | 4.188 | 4 |
| 500 | 5.235 | 5 |
| 600 | 6.282 | 6 |
| 700 | 7.329 | 7 |
| 800 | 8.376 | 8 |
| 900 | 9.423 | 9 |
| 1000 | 10.470 | 10 |
Because Minute of Angle is an angular measurement, you must know the distance before a linear measurement (size on a target) can be known/calculated.
Minute of Angle Explanation Video
If you’d like to see this in action and learn more, then be sure to check out the video I filmed for the NSSF.
How to Use Minute of Angle While Shooting
Now that we understand what MOA is, and how to calculate it, let’s look at how to use it when shooting.
The purpose of knowing Minute of Angle is so that you can make scope adjustments as the distance of your target changes. This will require some quick mental math, so let’s break that down and give some examples.
Here’s my Quick MOA calculation trick:
Think in Minute of Angle chunks for the distance you are shooting.
As an example, let’s pretend that you are shooting at 300 yards. From the MOA explanation above, we know that 1 MOA will equal about 3 inches at 300 yards. Therefore, start thinking in “3-inch chunks” for our 300-yard target. If I’m working with Mils, I just imagine centimeter-sized chunks.
If your bullet is 3 inches low at 300 yards, you know that 1 of your “3-inch chunks” will fit into that 3-inch adjustment. Therefore, you’ll need to adjust up to 1 MOA.
If however, your bullet’s impact is 6 inches low at 300 yards, then you should note that 2 of those “3-inch chunks” will fit within the 6-inch adjustment. Therefore, you would need to adjust up to 2 MOA at that distance.
Now, it doesn’t need to be even numbers to work. For example, with this method, you should be able to easily see that 1.5 MOA is needed to adjust 4 inches at 300 yards because 1.5 of those “3-inch chunks” will fit within the 4-inch difference.
This can also be useful when hunting. You can determine the diameter of a kill zone in MOA for a certain distance to know how far you’ll be able to shoot and make adjustments at different distances.
How Many Clicks are in an MOA?
Now that you know how many MOA you’d need to adjust in order to be more accurate with the target, the next question people ask is, “How many clicks on my scope should I make.”
The truth is, that depends on your scope. Some scopes need 4 clicks in order to change 1 MOA (called a 1/4 MOA scope). Some might require 2 clicks per MOA (1/2 MOA Scope). The key is to check your scope’s specs and know how many clicks equal 1 MOA as per your scope. However, the key is, if your scope is a 1/4 MOA, then it will need 4 clicks in order to change one MOA. Let’s do some examples:
Example 1: Your shot grouping is 1.5 inches right of target. How many clicks on a 1/4 MOA scope would you need to center your scope on the target?
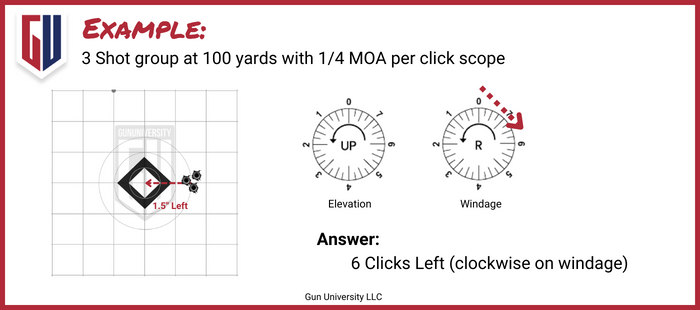
Example 2: Your shot grouping is 2 inches low, and 3 inches left of the target. How many clicks would you need with a 1/4 MOA scope in order to to zero in on the target?
Reminder: It is best to not think in clicks, but continue to think in MOA. This is because if you switch rifles and have a different MOA and if you stick with clicks, you’ll be confused.
MOA vs Mil
I’m often asked which is better, MOA or Mils? That really depends on a couple of things.
- What Scope are you using? If you’re scope is a MOA or MIL, then you’re best to study that particular one. Most hunting scopes are MOA, while most long range competitive scopes are in MIL
- Do you prefer Metric or Standard? As you can tell, MOA uses yards, where as MIL uses meters so which ever one you’re most comfortable using, should play into this.
I personally think in Minute of Angle because I’ve spent many years with it and have many MOA scopes.
For a more thorough comparison, please check out our article Mil vs MOA (Which is Best for You?)
Minute of Angle Formulas
Here are some formulas you can use when working with minutes of angle while shooting:
Simple MOA Formulas:
Yards to Target / 100 = inches per MOA adjustment at that distance
Inches of Adjustment Needed / Size of 1 MOA Chunk in Inches = Minute of Angle adjustment
Clicks per MOA on Scope x Number of MOA Adjustment Needed = Number of Clicks Needed for Adjustment
Note, if you want to use 1.047 inches per 100 yards instead of 1 inch per 100 yards, then multiply the (inches per MOA at that distance) by 1.047
MOA Calculations:
MOA -> Mils: MOA / 3.438 = Mils
MOA -> inches per 100 yards (rounded): MOA = inches per 100 yards
MOA -> inches per 100 yards (precise): MOA x 1.047 = exact inches per 100 yards
Mils -> centimeters per 100 meters: Mils x 10 = centimeters per 100 meters
To see a table with these calculations, check out the MOA table at the bottom of this article.
Quiz Time!
So as to make sure you’ve got this down, let’s go ahead and give a couple of questions and see how it goes.
How many Minute of Angle is 3 inches at 300 yards?
It is 1 MOA. Remember, because MOA is an angular measurement, its linear size gets larger the further away you go.
How many Minute of Angle is 9 inches at 600 yards?
It is 1.5 MOA. If you think in “chunks,” you know that 1 MOA is a 6-inch chunk at 600 yards. One and a half 6-inch chunks fit within the 9-inch size… therefore 1.5 MOA. So, if you measure that your bullet impacted 9 inches low at 600 yards, you’d adjust your turret up 1.5 MOA.
At 25 yards, how far will a bullet’s impact change with an 8 Minute of Angle adjustment?
2 inches. 1 MOA is 0.25 inches at 25 yards and 8 of those 0.25-inch chunks equal 2 inches.
If your scope adjusts 1/4 MOA per click, how many “clicks” are needed to move 2 inches at 100 yards?”
8 clicks. First, you should notice that I shouldn’t have talked in “clicks.” Good job. ???? Next you should first calculate how many Minute of Angle is needed – 2 MOA is needed to adjust 2 inches at 100 yards. Next, you should note that 4 “clicks” equal 1 MOA therefore 8 “clicks” are needed.
Long Range Shooting Handbook
This book is the complete beginner’s guide to long range shooting written in simple every-day language so that it’s easy to follow.
Included are personal tips and best advice from Ryan’s years of special operations sniper schooling and experience, and as a sniper instructor.
FAQ
Q: How big is a Minute of Angle at 100 yards?
A: A Minute of Angle (MOA) is about 1″ big at 100 yards. Precisely, is it 1.047″ big at 100 yards.
Q: Are Mil and Minute of Angle the same thing?
A: No, Mil and Minute of Angle are not the same things. However, they can be used in the same way as they are both angular measurements. “Mil” is short for Milliradian (1/1000th of a radian). To learn more about Mils, check out our Mils Guide.
Q: What is Minute of Angle used for?
A: Minute of Angle is used for adjusting a rifle scope either to sight in a rifle (zero) or to engage targets at different distances.
Q: Which can be adjusted on a scope more precisely (finely), MOA or Mil?
A: Minute of Angle can be adjusted in finer (smaller/precise) increments than Mils for most scopes. This is because most scopes that adjust in MOA adjust in 1/4 MOA per “click” whereas most Mil scopes adjust in 1/10 Mil per “click.” At 100 yards, 1/4 MOA is 0.25″ whereas 1/10th Mil is 0.36″
Q: How many clicks are 1 MOA increments on my scope?
If you have a Minute of Angle scope, it most likely adjusts in 4 clicks for 1 MOA increment. However, some scopes adjust in 1/2 MOA per click and some even have a turret that adjusts 1 full MOA per click. It’s important that you as the shooter know how much of an adjustment on your turret will move your bullet impact.
Minute of Angle Table
In this Minute of Angle table, we’ve given you easy conversions between MOA and Mils along with their sizes in inches and centimeters at different distances.
If you’d like to convert these numbers on your own, please see the Minute of Angle Formulas above.
| MOA | Mils | Inches/100 yds (rounded) | Inches/100 yds (exact) | CM per 100 meters |
|---|---|---|---|---|
| 0.25 | 0.07 | 0.25 | 0.26 | 0.73 |
| 0.5 | 0.15 | 0.5 | 0.52 | 1.45 |
| 0.75 | 0.22 | 0.75 | 0.79 | 2.18 |
| 1 | 0.29 | 1 | 1.05 | 2.91 |
| 1.25 | 0.36 | 1.25 | 1.31 | 3.64 |
| 1.5 | 0.44 | 1.5 | 1.57 | 4.36 |
| 1.75 | 0.51 | 1.75 | 1.83 | 5.09 |
| 2 | 0.58 | 2 | 2.09 | 5.82 |
| 2.25 | 0.65 | 2.25 | 2.36 | 6.54 |
| 2.5 | 0.73 | 2.5 | 2.62 | 7.27 |
| 2.75 | 0.80 | 2.75 | 2.88 | 8.00 |
| 3 | 0.87 | 3 | 3.14 | 8.73 |
| 3.25 | 0.95 | 3.25 | 3.40 | 9.45 |
| 3.5 | 1.02 | 3.5 | 3.66 | 10.18 |
| 3.75 | 1.09 | 3.75 | 3.93 | 10.91 |
| 4 | 1.16 | 4 | 4.19 | 11.63 |
| 4.25 | 1.24 | 4.25 | 4.45 | 12.36 |
| 4.5 | 1.31 | 4.5 | 4.71 | 13.09 |
| 4.75 | 1.38 | 4.75 | 4.97 | 13.82 |
| 5 | 1.45 | 5 | 5.24 | 14.54 |
| 5.25 | 1.53 | 5.25 | 5.50 | 15.27 |
| 5.5 | 1.60 | 5.5 | 5.76 | 16.00 |
| 5.75 | 1.67 | 5.75 | 6.02 | 16.72 |
| 6 | 1.75 | 6 | 6.28 | 17.45 |
| 6.25 | 1.82 | 6.25 | 6.54 | 18.18 |
| 6.5 | 1.89 | 6.5 | 6.81 | 18.91 |
| 6.75 | 1.96 | 6.75 | 7.07 | 19.63 |
| 7 | 2.04 | 7 | 7.33 | 20.36 |
| 7.25 | 2.11 | 7.25 | 7.59 | 21.09 |
| 7.5 | 2.18 | 7.5 | 7.85 | 21.82 |
| 7.75 | 2.25 | 7.75 | 8.11 | 22.54 |
| 8 | 2.33 | 8 | 8.38 | 23.27 |
| 8.25 | 2.40 | 8.25 | 8.64 | 24.00 |
| 8.5 | 2.47 | 8.5 | 8.90 | 24.72 |
| 8.75 | 2.55 | 8.75 | 9.16 | 25.45 |
| 9 | 2.62 | 9 | 9.42 | 26.18 |
| 9.25 | 2.69 | 9.25 | 9.68 | 26.91 |
| 9.5 | 2.76 | 9.5 | 9.95 | 27.63 |
| 9.75 | 2.84 | 9.75 | 10.21 | 28.36 |
| 10 | 2.91 | 10 | 10.47 | 29.09 |
| 10.25 | 2.98 | 10.25 | 10.73 | 29.81 |
| 10.5 | 3.05 | 10.5 | 10.99 | 30.54 |
| 10.75 | 3.13 | 10.75 | 11.26 | 31.27 |
| 11 | 3.20 | 11 | 11.52 | 32.00 |
| 11.25 | 3.27 | 11.25 | 11.78 | 32.72 |
| 11.5 | 3.34 | 11.5 | 12.04 | 33.45 |
| 11.75 | 3.42 | 11.75 | 12.30 | 34.18 |
| 12 | 3.49 | 12 | 12.56 | 34.90 |
| 12.25 | 3.56 | 12.25 | 12.83 | 35.63 |
| 12.5 | 3.64 | 12.5 | 13.09 | 36.36 |
| 12.75 | 3.71 | 12.75 | 13.35 | 37.09 |
| 13 | 3.78 | 13 | 13.61 | 37.81 |
| 13.25 | 3.85 | 13.25 | 13.87 | 38.54 |
| 13.5 | 3.93 | 13.5 | 14.13 | 39.27 |
| 13.75 | 4.00 | 13.75 | 14.40 | 39.99 |
| 14 | 4.07 | 14 | 14.66 | 40.72 |
| 14.25 | 4.14 | 14.25 | 14.92 | 41.45 |
| 14.5 | 4.22 | 14.5 | 15.18 | 42.18 |
| 14.75 | 4.29 | 14.75 | 15.44 | 42.90 |
| 15 | 4.36 | 15 | 15.71 | 43.63 |
| 15.25 | 4.44 | 15.25 | 15.97 | 44.36 |
| 15.5 | 4.51 | 15.5 | 16.23 | 45.08 |
| 15.75 | 4.58 | 15.75 | 16.49 | 45.81 |
| 16 | 4.65 | 16 | 16.75 | 46.54 |
| 16.25 | 4.73 | 16.25 | 17.01 | 47.27 |
| 16.5 | 4.80 | 16.5 | 17.28 | 47.99 |
| 16.75 | 4.87 | 16.75 | 17.54 | 48.72 |
| 17 | 4.94 | 17 | 17.80 | 49.45 |
| 17.25 | 5.02 | 17.25 | 18.06 | 50.17 |
| 17.5 | 5.09 | 17.5 | 18.32 | 50.90 |
| 17.75 | 5.16 | 17.75 | 18.58 | 51.63 |
| 18 | 5.24 | 18 | 18.85 | 52.36 |
| 18.25 | 5.31 | 18.25 | 19.11 | 53.08 |
| 18.5 | 5.38 | 18.5 | 19.37 | 53.81 |
| 18.75 | 5.45 | 18.75 | 19.63 | 54.54 |
| 19 | 5.53 | 19 | 19.89 | 55.26 |
| 19.25 | 5.60 | 19.25 | 20.15 | 55.99 |
| 19.5 | 5.67 | 19.5 | 20.42 | 56.72 |
| 19.75 | 5.74 | 19.75 | 20.68 | 57.45 |
| 20 | 5.82 | 20 | 20.94 | 58.17 |
| 20.25 | 5.89 | 20.25 | 21.20 | 58.90 |
| 20.5 | 5.96 | 20.5 | 21.46 | 59.63 |
| 20.75 | 6.04 | 20.75 | 21.73 | 60.35 |
| 21 | 6.11 | 21 | 21.99 | 61.08 |
| 21.25 | 6.18 | 21.25 | 22.25 | 61.81 |
| 21.5 | 6.25 | 21.5 | 22.51 | 62.54 |
| 21.75 | 6.33 | 21.75 | 22.77 | 63.26 |
| 22 | 6.40 | 22 | 23.03 | 63.99 |
| 22.25 | 6.47 | 22.25 | 23.30 | 64.72 |
| 22.5 | 6.54 | 22.5 | 23.56 | 65.45 |
| 22.75 | 6.62 | 22.75 | 23.82 | 66.17 |
| 23 | 6.69 | 23 | 24.08 | 66.90 |
| 23.25 | 6.76 | 23.25 | 24.34 | 67.63 |
| 23.5 | 6.84 | 23.5 | 24.60 | 68.35 |
| 23.75 | 6.91 | 23.75 | 24.87 | 69.08 |
| 24 | 6.98 | 24 | 25.13 | 69.81 |
| 24.25 | 7.05 | 24.25 | 25.39 | 70.54 |
| 24.5 | 7.13 | 24.5 | 25.65 | 71.26 |
| 24.75 | 7.20 | 24.75 | 25.91 | 71.99 |
| 25 | 7.27 | 25 | 26.18 | 72.72 |
| 25.25 | 7.34 | 25.25 | 26.44 | 73.44 |
| 25.5 | 7.42 | 25.5 | 26.70 | 74.17 |
| 25.75 | 7.49 | 25.75 | 26.96 | 74.90 |
| 26 | 7.56 | 26 | 27.22 | 75.63 |
| 26.25 | 7.64 | 26.25 | 27.48 | 76.35 |
| 26.5 | 7.71 | 26.5 | 27.75 | 77.08 |
| 26.75 | 7.78 | 26.75 | 28.01 | 77.81 |
| 27 | 7.85 | 27 | 28.27 | 78.53 |
| 27.25 | 7.93 | 27.25 | 28.53 | 79.26 |
| 27.5 | 8.00 | 27.5 | 28.79 | 79.99 |
| 27.75 | 8.07 | 27.75 | 29.05 | 80.72 |
| 28 | 8.14 | 28 | 29.32 | 81.44 |
| 28.25 | 8.22 | 28.25 | 29.58 | 82.17 |
| 28.5 | 8.29 | 28.5 | 29.84 | 82.90 |
| 28.75 | 8.36 | 28.75 | 30.10 | 83.62 |
| 29 | 8.44 | 29 | 30.36 | 84.35 |
| 29.25 | 8.51 | 29.25 | 30.62 | 85.08 |
| 29.5 | 8.58 | 29.5 | 30.89 | 85.81 |
| 29.75 | 8.65 | 29.75 | 31.15 | 86.53 |
| 30 | 8.73 | 30 | 31.41 | 87.26 |
| 30.25 | 8.80 | 30.25 | 31.67 | 87.99 |
| 30.5 | 8.87 | 30.5 | 31.93 | 88.71 |
| 30.75 | 8.94 | 30.75 | 32.20 | 89.44 |
| 31 | 9.02 | 31 | 32.46 | 90.17 |
| 31.25 | 9.09 | 31.25 | 32.72 | 90.90 |
| 31.5 | 9.16 | 31.5 | 32.98 | 91.62 |
| 31.75 | 9.24 | 31.75 | 33.24 | 92.35 |
| 32 | 9.31 | 32 | 33.50 | 93.08 |
| 32.25 | 9.38 | 32.25 | 33.77 | 93.80 |
| 32.5 | 9.45 | 32.5 | 34.03 | 94.53 |
| 32.75 | 9.53 | 32.75 | 34.29 | 95.26 |
| 33 | 9.60 | 33 | 34.55 | 95.99 |
| 33.25 | 9.67 | 33.25 | 34.81 | 96.71 |
| 33.5 | 9.74 | 33.5 | 35.07 | 97.44 |
| 33.75 | 9.82 | 33.75 | 35.34 | 98.17 |
| 34 | 9.89 | 34 | 35.60 | 98.89 |
| 34.25 | 9.96 | 34.25 | 35.86 | 99.62 |
| 34.5 | 10.03 | 34.5 | 36.12 | 100.35 |
| 34.75 | 10.11 | 34.75 | 36.38 | 101.08 |
| 35 | 10.18 | 35 | 36.65 | 101.80 |
| 35.25 | 10.25 | 35.25 | 36.91 | 102.53 |
| 35.5 | 10.33 | 35.5 | 37.17 | 103.26 |
| 35.75 | 10.40 | 35.75 | 37.43 | 103.98 |
| 36 | 10.47 | 36 | 37.69 | 104.71 |
| 36.25 | 10.54 | 36.25 | 37.95 | 105.44 |
| 36.5 | 10.62 | 36.5 | 38.22 | 106.17 |
| 36.75 | 10.69 | 36.75 | 38.48 | 106.89 |
| 37 | 10.76 | 37 | 38.74 | 107.62 |
| 37.25 | 10.83 | 37.25 | 39.00 | 108.35 |
| 37.5 | 10.91 | 37.5 | 39.26 | 109.08 |
| 37.75 | 10.98 | 37.75 | 39.52 | 109.80 |
| 38 | 11.05 | 38 | 39.79 | 110.53 |
| 38.25 | 11.13 | 38.25 | 40.05 | 111.26 |
| 38.5 | 11.20 | 38.5 | 40.31 | 111.98 |
| 38.75 | 11.27 | 38.75 | 40.57 | 112.71 |
| 39 | 11.34 | 39 | 40.83 | 113.44 |
| 39.25 | 11.42 | 39.25 | 41.09 | 114.17 |
| 39.5 | 11.49 | 39.5 | 41.36 | 114.89 |
| 39.75 | 11.56 | 39.75 | 41.62 | 115.62 |
| 40 | 11.63 | 40 | 41.88 | 116.35 |
| 40.25 | 11.71 | 40.25 | 42.14 | 117.07 |
| 40.5 | 11.78 | 40.5 | 42.40 | 117.80 |
| 40.75 | 11.85 | 40.75 | 42.67 | 118.53 |
| 41 | 11.93 | 41 | 42.93 | 119.26 |
| 41.25 | 12.00 | 41.25 | 43.19 | 119.98 |
| 41.5 | 12.07 | 41.5 | 43.45 | 120.71 |
| 41.75 | 12.14 | 41.75 | 43.71 | 121.44 |
| 42 | 12.22 | 42 | 43.97 | 122.16 |
| 42.25 | 12.29 | 42.25 | 44.24 | 122.89 |
| 42.5 | 12.36 | 42.5 | 44.50 | 123.62 |
| 42.75 | 12.43 | 42.75 | 44.76 | 124.35 |
| 43 | 12.51 | 43 | 45.02 | 125.07 |
| 43.25 | 12.58 | 43.25 | 45.28 | 125.80 |
| 43.5 | 12.65 | 43.5 | 45.54 | 126.53 |
| 43.75 | 12.73 | 43.75 | 45.81 | 127.25 |
| 44 | 12.80 | 44 | 46.07 | 127.98 |
| 44.25 | 12.87 | 44.25 | 46.33 | 128.71 |
| 44.5 | 12.94 | 44.5 | 46.59 | 129.44 |
| 44.75 | 13.02 | 44.75 | 46.85 | 130.16 |
| 45 | 13.09 | 45 | 47.12 | 130.89 |
| 45.25 | 13.16 | 45.25 | 47.38 | 131.62 |
| 45.5 | 13.23 | 45.5 | 47.64 | 132.34 |
| 45.75 | 13.31 | 45.75 | 47.90 | 133.07 |
| 46 | 13.38 | 46 | 48.16 | 133.80 |
| 46.25 | 13.45 | 46.25 | 48.42 | 134.53 |
| 46.5 | 13.53 | 46.5 | 48.69 | 135.25 |
| 46.75 | 13.60 | 46.75 | 48.95 | 135.98 |
| 47 | 13.67 | 47 | 49.21 | 136.71 |
| 47.25 | 13.74 | 47.25 | 49.47 | 137.43 |
| 47.5 | 13.82 | 47.5 | 49.73 | 138.16 |
| 47.75 | 13.89 | 47.75 | 49.99 | 138.89 |
| 48 | 13.96 | 48 | 50.26 | 139.62 |
| 48.25 | 14.03 | 48.25 | 50.52 | 140.34 |
| 48.5 | 14.11 | 48.5 | 50.78 | 141.07 |
| 48.75 | 14.18 | 48.75 | 51.04 | 141.80 |
| 49 | 14.25 | 49 | 51.30 | 142.52 |
| 49.25 | 14.33 | 49.25 | 51.56 | 143.25 |
| 49.5 | 14.40 | 49.5 | 51.83 | 143.98 |
| 49.75 | 14.47 | 49.75 | 52.09 | 144.71 |
| 50 | 14.54 | 50 | 52.35 | 145.43 |
Subscribe To Our Newsletter
Join our mailing list to receive the latest news and updates from our team
About Ryan Cleckner
Ryan is a former special operations sniper (1/75 Ranger) and current firearms attorney, firearms industry executive, university lecturer, and bestselling author of the Long Range Shooting Handbook.
Recent Posts
November 22, 2024
November 22, 2024
November 21, 2024
November 20, 2024
What is the relationship between the optic MOA rating and the value in MOA/click on the adjustment screws on a red dot?
Ryan
This is an over complication of the issue, in your explanation of MOA you show that one MOA is 1/60th of one degree in a 360 degree circle (The “Angle” in MOA (Minute of Angle) is one of the 360 degrees in a circle. Therefore, MOA is 1/60th of one degree in a circle.), is it correct to deduce that there 21,600 MOA’s in a full circle.
Like I said “over complication”.
Enjoyed your video and written explanation.
Thank you for your SERVICE.
Excellent,well done
Thank You Sir.
Ryan,
Do you have a Long range shoot school? I would like to come to a class and learn from you in person. If so, can you sent me your link to get more information on MOA course and how to be a better long rang shooter.
Ryan,
What will the total MOA of one bore sighting shot at 25 yds, impacting the target 3 in. left of bullseye, and then using this same target at 100yds using the previous impact shot to determine the 100yd MOA. Do I combine both MOA’s?
Edward, you’ve lost me. At 25 yards, 3 inches left would mean that barrel is pointed 12 MOA left of the reticle. Without changing anything other than shooting at 100 yards, it would be 12″ to the left. At 1000 yards (with no wind or spin drift) it would be 120″ to the left.
If the 25 yard shat was a good one, I would adjust 12 MOA right, and 4 MOA down (I want to be an inch low at 25 yards). Then, I’d shoot a group on paper at 100 yards and makes adjustments from there.
This was a great training aid. Quick question, if I’m zeroed in at 100 yards. Would it still be 2″ at 200 yards? Or would it now be 1″? I normally set my scope where I’m shooting 1″ high at 100yards. And it tends to be dead on at 200 yds. Asking because I hunt a farm where there are multiple distances and most times you haven’t the time to adjust your MOA. So I tend to aim high insted of adjusting my elevation. I guess what I’m getting at, if I’m zeroed in at 100 yards and if I had to make a shot at 400 yards would my MOA now be 3″ or would it still be a 4″ adjustment. Thank you Bud.
I’m happy to have helped.
However, I may not have explained it well.
MOA is just a unit of measurement and it is not tied to any particular cartridge or rifle. For example, in your question about “what you MOA would be” at 400 yards, there’s no answer for that without knowing your cartridge’s ballistics.
If your bullet drops 36 inches at 400 yards (IF), then you would need to adjust up 9 MOA (36/4=9).
Likewise with your 200 yard question. Your bullet drops however much it drops. Then, you can calculate how much MOA to adjust to compensate for that drop at a certain distance. If your bullet drops 2″ at 200 yards with a 100 yard zero, then you’d adjust up 1 MOA at 200 yards (which is 2″).
This is more of a question about your great video. You said that the 308 will drop a bullet at the following distance- 200 yards 4 inches, 300 15, 400 32, 500 60. When you do scope adjustment do you need to know the bullet drop to do MOA?
also, how did you get those numbers and what would they be for 600-2000 yards?
This is the best explanation of MOA I have ever read. I was having some trouble with MOA until I stumbled on your explanation, video and book Thank you very much.
Thanks for the kind words! I’m happy to have helped you and I appreciate your support.